Hilbert’s curve…

Sometimes I like to look at how math creates patterns to see if there is something original I could work with to create a new design.  In 1891, or thereabouts, a German named David Hilbert documented a fractile, or a product that divides into equal parts, that creates a pattern.  Basically, this is a mathematical function that will create a pattern, but we’re not going to do any math, we’re just going to look at the resulting pattern…

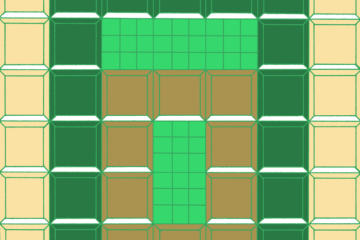
If you can draw a “U”, draw the same “U”, and then rotate that “U” twice 90 degrees each time, the four different “U”s become Hilbert’s curve, which is one of many “space filling patterns” that can be created via math.  Undergraduates are sometimes tasked with identifying new space filling patterns.  (Hey, it’s not such a bad fate to get something named after you!)

When I looked at Hilbert’s curve, I thought about how I create patterns with my motifs by rotating them 90 degrees and wondered about multiple rotations…  this is an interpretation of Hilbert’s curve, and I like the result!  Food for thought…